You will find the basic facts about Plastic-Frictional Theories (Part. 3) - no details -. Detail is a matter of my current Ph.D. research and I will not show that here. If you wanna know more just email me or feel free to ask in the Volcano Discussion Forum. This general overview should help you to understand the modeling results and their interpretations that will be presented in this Granular Volcano Group Web Site. I purposely erased all the bibliographical references and detailed equations to keep the text simple and easy to read.

If you have reached this page, please, be aware that this whole site may be better seen at the following urls:

http://www.granular-volcano-group.org
or
http://www.granular.org

Those new urls will lead you to a faster, non-commercial, and pop-up free website. These are our official url addresses. Please, update your bookmarks.

If you wish, you may see this specific page on the new website here:
Part. 3 - Critical State Theory

__________________________

We have seen on the preceding sections:
I. Introduction
II. Stress space, Slip Planes, Mohr-Coulomb and von Mises stresses
      II.1. Mohr-Coulomb case: a 2D representation of stress (particular case)
      II.2. von Mises case: a 3D representation of stress (general case)
III. Plastic Potential Theory

On this page, you will find:
IV. Dilatation, consolidation, yield locus, and critical state
      IV.1. Dilatancy
      IV.2. Consolidation
      IV.3. Yield locus and critical state

And on the following page, you will find the continuation of this frictional course:
V. Constitutive Equations for Frictional Granular Flows
      V.1. Beyond the extended von Mises Yield locus
      V.2. Within the extended von Mises Yield locus

Please, don’t forget to sign our Granular Volcano Guest Book.

Done and updated by Sébastien Dartevelle, The WebMaster, June 29th, 2002.

IV. Dilatation, consolidation, yield locus, and critical state

Let’s imagine we could perform the following idealized experiment (see Figure 10). Between two rough (no-slip) plane stands a granular material. The bottom plate is immobile while the upper plate can move laterally to generate a shear stress (S) within the granular medium, hence the material deforms (shear strain, ) as the plate moves. The whole material has a uniform bulk density () and is under a constant load N (normal stress) on the upper plane. Again, I will assume -as I have done so far- that the granular material is plastic-rigid (i.e., it does not show any elastic deformation under applied stress) and cohesionless.

They are two different behaviors of the granular medium that can be observed depending on the normal loading (N) and/or the initial bulk density (): dilatation and consolidation.

[Back to Top]

IV.1. Dilatancy

For high density and/or low normal pressure, the behavior is called failure, dilatation, or dilatancy of the material and is shown on Figure 11. At first, as the shear stress increases, no noticeable shear deformation occurs (except maybe a reversible elastic strain that I do not show on Fig. 11A), till we reach a critical shear stress (S_c) which depends on the applied normal stress and the bulk initial density (Fig. 11A). Afterwards, instead of having a flat linear line of S vs. , the required applied shear stress may be asymptotically decreased to some constant value (S_inf) independent of the bulk density and only a function of the normal stress. Fig. 11B shows the displacement of the granular material as a function of the position of the plates (only the upper plate moves). Typically the shear strain occurs in a very thin layer, while the upper part of the flow moves en bloc without no noticeable shear within it.

This behavior is described as a failure of the granular material. The decrease of the value of S needed to maintain shear after passing the failure point (S_c) is associated with a weakening of the material during the initiation of the plastic deformation (basically, we need less shear force for deforming the material). In the thin shear zone, we would measure a decrease of the bulk density owing to an overall dilatation of the shear layer. This dilatation phenomenon arises from the need of a densely packed granular material to spread in order to make enough room for allowing grains to move.

In the plane or in the S-N plane (it doesn’t matter which plane because , since according to Mohr-Coulomb, we have ), for a given initial bulk density and at failure (or at yield), we would have a convex curve as shown on Fig. 11C. This kind of curve is called failure locus or yield locus. So, the higher the normal stress N, the higher the shear stress S for reaching failure. Also, there is a different failure locus for different initial bulk densities. Typically, for a given normal stress, the higher the bulk density, the higher S required for reaching yield. It should be noted that once values of S asymptotically tend to S_inf, the material tends to a constant density (Fig. 11A), and therefore the dilatation of the material ceases.

You may be surprised to see curved yield loci as we would have expected rather a straight line in the or in the S-N plane as predicted by the Mohr-Coulomb Law. Yeah, that’s true but as I said earlier the Mohr-Coulomb is only an ideal state which may not be quite right. In addition, the curvature of those yield loci is very low, and at the limit those curves can be approached by a straight line. However, for some materials, the curvature may be significant and a simple straight Mohr-Coulomb line is indeed inappropriate (then a Warren Spring Failure locus curve should be used instead). Generally speaking, for a given yield locus, the curvature is only pronounced at low normal stress.

[Back to Top]

IV.2. Consolidation

When is small and/or N large, the situation is totally different (Figures 12). Again, we need to reach a critical value of the shear stress (S_c) to initiate deformation (Fig. 12A). Then afterwards, shear deformation starts but to maintain it, we must asymptotically increase the applied shear stress to a constant value (S_inf), independent of the bulk density. In Fig. 12B, it can be seen that, in this case, the shear strain is distributed uniformly throughout the granular layer. In addition, we would measure a uniform increase of the bulk density in the whole layer as deformation proceeds. However, once S_inf is approached, the granular material tend to a fairly constant bulk density, hence consolidation ceases.

In this case, the material gains strength since to initially maintain the deformation we must increase the applied shear stress. The increase of bulk density of the flowing material can be seen as the upper plate moves downwards. This phenomenon is called consolidation, contractancy or negative dilatancy. In the or S-N plane, for a given initial bulk density and at yield, we would have the convex curve as shown on Fig. 12C, where the higher the normal pressure, the less shear stress we need to initiate consolidation. As for the dilatation, those curves in the /S-N plane depend on the initial bulk density. For a given normal stress, the higher , the higher the shear stress for reaching yield. Such a kind of curve in the plane is named consolidation locus or contractancy locus. Once the critical shear stress is reached (S_c), the shear stress asymptotically tend to a fairly constant value (S_inf). Notice that for very high value of normal stress, , where the consolidation surface intersect the N-axis (Fig. 12C). At such intersection point, the material consolidate under normal load alone, without any assistance from shear stress.

[Back to Top]

IV.3. Yield locus and critical state

As we have just seen the yield locus (e.g., failure locus and consolidation locus) is a function of the bulk density () and the normal stress. For a given density and for a 2D stress representation, the function yield locus , is convex as shown on Fig. 11C & 12C and Figures 13 in the /S-N plane. In Figure 13A, where I drew both the failure locus and the consolidation locus, we can interpret such a curve as a section of a surface in a space. In soil mechanics, the surface that represents the failure part is called the Hvorlsev Surface, while the consolidation part in this space is named Roscoe surface. The material is rigid if is less than the threshold and is perfectly plastic on the curve. As mentioned above, the higher the initial bulk density of the granular material, the higher its strength (we need to apply more normal and/or shear stress for deforming it) as seen on Figure 13. In the space, the intersection of the Roscoe and Hvorlsev Surfaces defines a curve called the Critical State Locus. This curve projected onto the plane defines a curve called the Termination Locus , which is most of the time a straight line. This straight line intersect the yield loci at a point named critical state. (Fig. 13A & 13B). Some authors give a supplementary property of the critical state: on a given yield locus curve (i.e., for a given bulk density), the critical state is where the derivative of vanished, in other words: (Fig. 13A). The material will consolidate or expand according to whether () lies to the right or left side of the line of critical states. Hence, we have on this yield locus an expansion side (left side, where ) and a consolidation side (right side, where ) (see Fig. 13B). This means that when the granular material reaches the critical state point on a given yield locus, it will deform without any change of its volume ( ). Since, at yield, the granular material suffers density change, it implies that the granular material, as it is deforming, will move from one yield locus curve to another until it finally reached the critical state where it will deform without any further change of volume.

I guess you have notice that the notion of "yield locus" is somehow ambiguous as it refers to two different things. Yield locus can be used as failure locus and therefore represents the left side of the whole curve drawn on Fig. 13A (the side that could be represented by a Mohr-Coulomb Law). However, some authors used yield locus for describing the whole curve, that is failure locus curve (left-side) and consolidation locus curve (right-side). So, you must be very careful of the context in which "yield locus" is used within the text (I know it is horribly confusing …).

Let’s go back to Fig. 13C and see what happens to a granular material of initial bulk density, , subject to normal stress smaller or greater than the normal stress at its critical state. If the normal stress is greater than the normal stress defined by the critical state (right-side), then we will have a consolidation behavior (path X-Y-Z). In this case the granular material is initially at point X, and will not suffer any strain as long as the shear stress is smaller than the threshold value defined by the point Y on its consolidation curve . As soon as the shear stress reached that particular value at the point Y, the granular material will plastically compress and strengthen until it reaches a termination locus (critical state point) in Z. This material has moved from an initial density to a higher density . Thereafter, the material will continue to shear at constant bulk density. Because, the material has increased its density, this behavior is named consolidation. Now, if the normal stress is smaller than the critical state normal stress (left-side), we will have a dilatation behavior (path Q-R-S). The material is initially at Q, and will not suffer any strain as long as the shear stress is smaller than a threshold value of shear stress defined by the point R on its failure curve . As soon as the material is on that curve, at R, it will plastically shear and dilate and therefore weaken until it reaches a termination locus at S. This material has decreased its initial density from to , this behavior is named failure or dilatancy. The dilatation occurs in very confined local zones which most of the time has already been previously sheared (weak zone).

All this can be also thoroughly analyzed in the stress plane, it is exactly the same story. In this plane, yield locus may have the shapes as indicated in Figure 14A which also shows the directions of the normal to the locus in three different point: 1, 2, and C. At point 1, for low values of and hence for low value of normal stress, the normal vector has a positive projection on the line of slope 45° (which is the isotropic normal Pressure defined as 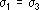). Yielding in this particular case is accompanied by dilatation. At point 2, for high values of (hence high value of normal stress) the normal vector has a negative projection on the isotropic normal Pressure line, and yielding is characterized by a compaction. It is easy to see from Fig. 14A, that the point C is the critical state where the material at yield has no change in volume since the normal is perpendicular to the isotropic normal stress line. In Fig. 14A, we have the yield locus for only one value of the bulk density. In Fig. 14B, the same figure is constructed for three different bulk densities and the lines of critical states is also shown. Different situations are represented (see trajectories Q-R-S for dilatation and X-Y-Z for consolidation, the same situation as Fig. 13C). If yield is initiated when the granular material is just at point Q, dilatation must occur, and if the normal stress is maintained constant, then the material will loose its bulk density (following the path Q-R-S) till it reaches the critical state at point S, where deformation will proceed without any change in density. On the other hand, if yield is initiated at a point beyond the critical state (for high values of normal stress at position X), then consolidation will occur. The material is gaining strength and it sees its bulk density increasing. For a constant normal stress, it follows the path X-Y-Z till it reaches the critical state in Z. Again we have the same story as Fig. 13C, but I simply wanted to show you this from another "stress perspective" (the more the better).

Hope all this makes sense for you, at least it does for me. There is a last thing I’d like to say, hoping you wont be more confused than ever ? What about the von Mises and Mohr-Coulomb Laws in all this? Well, If you remember what I said in the Plastic Potential Theory page (Part. 2 of this course): those two laws seem to predict dilatation (failure) of the granular material. Well, for instance, in the Mohr-Coulomb case it is clear that the failure locus in Fig. 13 (left-side) can be approximated by a straight-line. Hence, the Mohr-Coulomb law cannot predict neither the consolidation, nor the critical state. In other words, the Mohr-Coulomb law is only a failure locus. And this is also the case in 3D in using a conical extended von Mises yield locus, which only predicts dilatancy process since the projection on the hydrostatic axis of the normal to the yield locus is negative (see Fig. 9 in the Part.2 of this course). Therefore, the Mohr-Coulomb and von Mises yield loci can only predict dilatation process. If you use those laws, you wont be able to approach the true phenomena seen in granular maters. Those two laws may be OK for some cases but you should think twice before using those laws.

So, now, what I am gonna do is to develop constitutive equations … I will also suggest a possible more general and useful law than the Mohr-Coulomb/von Mises one. All this can be found in the last Part of this course:

~~~~~~~~~ Part 4: V. Constitutive Equations for frictional granular flow ~~~~~~~~~

If you have enjoyed this Plastic-Frictional (Part 3) webpage, pleaaase, before you leave, sign my Guestbook. It's all I ask!

[Back to Top]

You may also enjoy those pages:

| Guestbook | Discussion Forum | Chatroom | Volcano Links | Granular Links | Awards | Logos | Who is the Webmaster? |

_____________________________________________________

Any problems or comments with this web site can be reported to the Web Master.