We know that the slope of the curve is given by

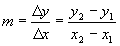
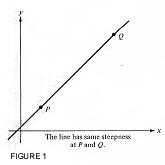
Where (x1,x2) and (y1,y2) are any two points on the line. Thus the slope of a line is a constant, (Figure 1). This is not the case for other curves. For instance, in (Figure 2) it appear that the curve is steeper (has a greater slope) at point Q than at point P. This suggests that for nonlinear curve the slope is not constant, but actually varies from point to point. In this lecture we introduce a procedure for determining the slope of a curve at a point.

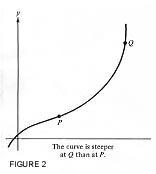
To determine the slope of a curve at some point, we make use of the tangent line to the curve at the point. For instance, in (Figure 3) the tangent line to the graph of ƒ at P is the line that best approximates the graph of ƒ at that point. Our problem of finding the slope of a curve at a point thus becomes one of finding the slope of the line tangent to the curve at that point.

Recall that the familiar "tangent line to a circle" required the circle and the line to have only one point on common. Such is not the case in (Figure 3), as the dotted portion of the tangent line indicates. Informally,when we refer to a tangent line to a curve, we mean a local tangent to the curve at a specific
point and we do not care if the line and the curve intersect at some other point.

We can identify the tangent line at a point in the following manner. Consider the secant line through the two points P and Q on the graph of y=ƒ (x) in (Figure 4). Suppose point Q moves along the curve towards P,determining the secant lines from point P to Q, from P to Q1, and so on. Now as Q moves closer and closer to P, observe that these secant lines approach a limiting position. This limiting position of the secant liness is called the tangent line to the curve P.

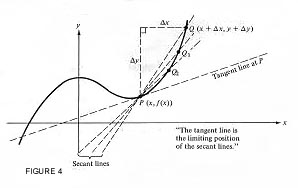
Now to determine this tangent line at P, we need to know its slope. Since the tangent line is the limiting position of the secant lines, its slope is the limiting value of the slopes of the secant lines as Q approach P. Informally, this can be represented by:

From (Figure 4),we see that the slope of the secant line through P and Q is

As QP, we have x0. Therfore, the slope of the tangent line

We are now ready to define the slope of a curve at a point on the curve.

To calculate the slope of the tangent line to a curve by its limit definition, we follow general four-step

Four-Step Process                                             Given y=ƒ(x):                                                     1) Determine ƒ(x+x).                                                     2) Calculate ƒ(x+x)-ƒ(x).                                                     3) Divide by x to obtain                                                                                                                         4) Let x0 to obtain

Since the derivative ƒ'(x) and the slope of the tangent line to the graph of ƒ are both defined by the limit.

We can use the four-step process to determine ƒ'(x).