BECAUSE GALAXIES' REDSHIFT IS CAUSED BY "LIOU_SCHWARTZ'S STRETCH EFFECT" NOT BY "DOPPLER'S EFFECT".
There are only three different kinds of spaces, Euclidean, Elliptic and Hyperbolic. That's mean there are three different kind of Universe. Most astronomer agree that the Universe is not a Euclidean. Assume we are in Elliptic Universe. All the light ray is a geodesic. Light ray will circle the geodesic forever. We are not only can see the same star many times in one direction, we also can see the same star many times in opposite direction. This is not the case. Another proof of not been Elliptic Universe is, the Universe had been so many billions of years. When light ray circle the geodesic forever. It will not escape from our Universe. When collect so many billions of years. Our Universe will be so bright that even in nighttime. Of course this is not the case neither. So our Universe should not be Elliptic Universe. The only possibility is Hyperbolic Universe.
If we are in Hyperbolic space. Very logically all the astronomy data should use the Hyperbolic formula and rules. This is how author concludes that the redshift of Galaxies are cause by the nature of Hyperbolic space, in which the space stretches the light spherical front.
A.
WHAT
KIND OF UNIVERSE IS WE IN? EUCLIDEAN, ELLIPTIC
OR
HYPERBOLIC UNIVERSE.
There are only three kinds of space Euclidean , Hyperbolic or Elliptic.
Almost all astronomy agrees that the Universe is not Euclidean space. The only two lefts are Hyperbolic and Elliptic.
In
Elliptic space, every light ray is a geodesic line. Light rays will be circling
the geodesic line forever unless blocked by some object. We can see the same
light ray of star many times, because the light ray circles in the space forever.
Also, we can see the same star from opposite directions many times, because the
light ray from other side of star circles in the space forever too. Since
the Universe was at least many billions old. All the lights of the sun and
stars had collected so many billions of years. The Universe should be so bright
even at nighttime. Of course that is not the case. So the possibility of the
Universe is only Hyperbolic Universe.
Hubble's
laws are derived from Euclidean rules and Euclidean formulas. However, if we
assume the Universe is in Hyperbolic space, very logically, we must derive its
rules and formulas from Hyperbolic rules and Hyperbolic formulas.
The
rules and formulas of Euclidean and Hyperbolic mathematics are quite different.
Hence, the results derived from utilizing these two systems must be different.
These differences may be the keys to unveil the mystery of the Universe.
B.
SPHERICAL WAVE FRONTS
When
a photon travels a distance 
.
The equation of a light spherical front in Euclidean space is
-------------- (1)
From
Hyperbolic geometry, the equation of the light spherical front is
-------------- (2)
Where
k is the constant of the space
curvature.
(From
page 298 of non-Euclidean Geometry by Allen Liou, 1964.)
Comparing
equation (1) and (2), we can see that the area of the light spherical fronts is
very different. Therefore, the Doppler Effect should not be the same for
applied between Euclidean space and Hyperbolic space.
The
area of the Light Spherical Front in Euclidean space is 
.
What
is the area of the Light Spherical Front in Hyperbolic space ?
Let
us determine the circumference of a circle in Hyperbolic space:
Let
PQ be the chord of a circle of radius 
, which subtends an angle 
, M be the midpoint of the chord, and O be the center of the
circle.
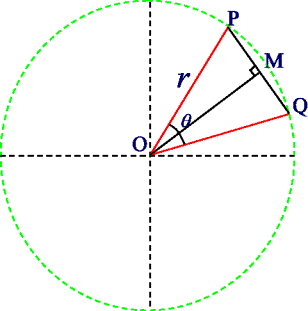
From
the formula of the right-angle in Hyperbolic trigonometry, we have (page 143 of
non-Euclidean Geometry by Allen Liou, 1964.)
If
angle 
à 0
or 
Integrating
both sides, we have
Circumference = 
Then,
let 
be the length of the
arc of the spherical circle, and 
be the radius.
By
same formula, we have
The
area of the circle strip is
But
Therefore
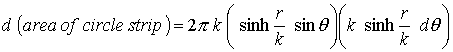
Integrating
both sides, we have
C.
DOPPLER EFFECT OR "LIOU'S STRETCH EFFECT"
When
a photon travels a distance 
,
the area of the Light Spherical Front in Euclidean space is 
.
But the area of the Light Spherical Front in Hyperbolic space is
Comparing
the two Spherical Areas in the two different spaces, we easily see that, if we
are in Hyperbolic universe, the Light Spherical Front stretches from 
to 
. We temporarily call this "Liou's stretch effect".
The
photon may only travel a distance 
in Hyperbolic space.
But in Euclidean space, it appears to travel a distance of 
. When 
is large enough, 
is much larger than 
.
From
the difference of
and 
in Euclidean space,
it looks like the object moves from point 
to point 
, but the object actually stays still in Hyperbolic universe.
From this fact, we can use the Doppler effect in Euclidean universe to
calculate galaxy movement away from the Earth as a result of the Universe's
expansion or use "Liou's stretch effect" in Hyperbolic universe to calculate
the constant of space curvature. Redshift of Doppler effect is caused by the
velocity of a moving object. Redshift of
"Liou's stretch effect" is caused by the nature of Hyperbolic space. It
is static not moving.
Since
I have only the data of Hubble's constant in velocity not the redshift of
frequency. I will use the velocity to calculate the space constant. Using
redshift of frequency the result is the same.
D.
CALCULATION OF SPACE CURVATURE (OR COSMOLOGICAL CONSTANT) IN HYPERBOLIC
UNIVERSE
Let s = 
Where
s is the distance of galaxies look
like moving from point 
to point 
in Euclidean space.
Taking
the derivative of both sides, we have
where
=v (the velocity of
galaxies at the remote distance of r),
and 
is the speed of light
c.
Therefore
-------------
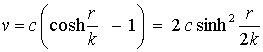(3)
There
are several versions of the Hubble's constant. We will select the one most
popular one in which, the velocity of galaxies at a distance of six billion
light-years move away at a velocity of roughly 90,000 kilometers/sec.
Hence
v=90,000 kilometers/sec and r=6 bly.
Hence
we have
90,000 = 
where
bly is billion light-years.
D.
DISCUSSION
1. Hubble's constant was not constant.
From
equation (3), the velocity of galaxies and the remote distance of r were not exactly linear proportions in
Euclidean universe. The velocity is more likely in slightly acceleration
observed in Euclidean universe.
Here,
the cosmological constant, 
, was based on the Hubble's Law at 6 bly. If we based our calculations on a different distance, like one
on a distance of 1 or 2 bly, the k value should be slightly different. If
we use different versions of Hubble's Law, the cosmological constant k will be even more different. We really
need accurate data to determine the constant k.
Assuming
is correct, the Hubble's diagram in Euclidean space should look
like the following diagram.
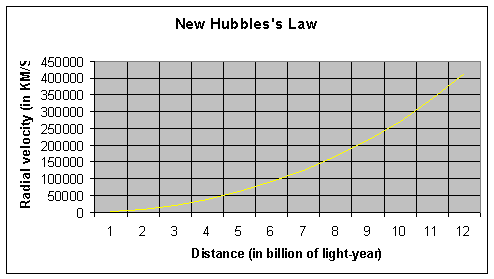
From
this chart, it should be called Hubble's accelerator instead of Hubble's
constant.
2. What
kind of Universe we are really in? Euclidean, Elliptic or Hyperbolic Universe.
There
are only three different kind of space, Euclidean, Elliptic or Hyperbolic space.
From the evident we have so far most agree it is not a Euclidean space. The
only two spaces left to chose are Elliptic or Hyperbolic space.
Assume
Elliptic Universe is true. What we should see and predict?
In
Elliptic Universe, every light ray is a geodesic line. That mean the light ray
will circle the geodesic circle forever unless block by some objects. That mean
we are not only able to see the same star many times in one direction, also we
can see the same star in opposite direction many times. More over, since the
Universe had many billions years old, Universe collect so many billions years of
lights. Every light is circling forever. What result of this will be the light
all day and all night. Of cause, that is not the case, so the most possibility
of the Universe is Hyperbolic Universe.
3. Is
Universe's redshift cause by DOPPLER
EFFECT or "LIOU'S STRETCH EFFECT"?
From
Hubble's Law, the speed by which a galaxy moves away is proportional to the
distance to the galaxy. A galaxy with
distance of 6 bly has a velocity of
90,000 km/s. For a galaxy 30 bly away, its speed will be 450,000
km/s. This is beyond the speed of light. It is contradiction to the fact of the speed of light is constant.
In
recent years, astronomers observed that Hubble's constant is not constant. The
galaxies moving away actually accelerated. This coincides with the prediction
from the chart of new Hubble's constant above.
From
these two facts, the Universe's redshift is more likely to be caused by the
"LIOU'S STRETCH EFFECT".
.
4.The accuracy of the cosmological constant
k, not only depends on the accuracy of Hubble's Law; it also depends on the
distance between galaxies. However, the distance between galaxies may have to
be reconstructed.
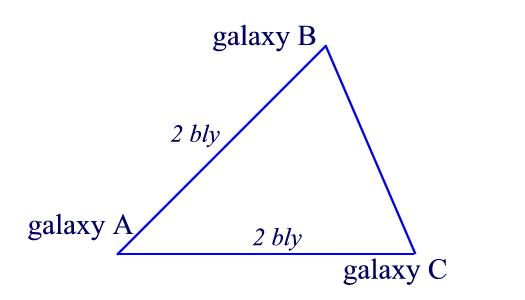
For
example, let us consider three galaxies A,
B and C, with 
; 
.
In
Euclidean Universe, distance BC will
be
-------------- (4)
In
Hyperbolic Universe, distance BC will
be, as follows
(from
page 150 of non-Euclidean Geometry by Allen Liou, 1964.)
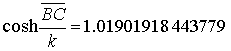
-------------- (5)
Comparing (4) and (5), we know (5) > (4). Obviously, the computations in Euclidean and Hyperbolic spaces are quite different.
In
my opinion, "LIOU'S STRETCH
EFFECT" in the
Hyperbolic Universe with curvature 
is more reasonable
than the DOPPLER EFFECT in the Euclidean Universe. If there is no expansion of
the Universe, there is no Big Bang.
Before
1930, Einstein's view was that the Universe was static with a "cosmological
constant". When Hubble introduced the idea that the Universe was expanding,
Einstein dropped the idea of cosmological constant and called it "the greatest
blunder of my life".
Perhaps
Einstein should not have dropped the idea of a cosmological constant so
quickly. If he believed that the Universe was in Hyperbolic space, then there
is a space curvature which is the cosmological constant.
After 1930, Hubble showed that the Universe was expanding. Besides the Big Bang theory, there are a dozen versions of the inflationary theory. The concept is rather complicated and not very promising. In my opinion, the best inflationary theory is one with no inflation. The best concept should be simple, clear, easy to calculate, and accepted by most people.
I
don't know how astronomers calculate the distance of galaxies. For accuracy,
maybe we need to use Hyperbolic rules and formulas to calculate the distances
between galaxies. Perhaps all the cosmological data should be calculated using
Hyperbolic rules and formulas.
1. Allen C. Liou 1953 "Non-Euclidean Geometry"
2. Baade, W., and Hubble, E. 1939, Pub. Astron.
Soc. Pac., 51, 40.
3. Hubble, E. 1934b, Redshifts in the Spectra of
Nebulae, (Halley Lecture), (Oxford: The Clarendon Press.).
4. Two methods of investigating the nature of nebular red-shift - Edwin
Hubble and Richard C. Tolman, Bibcode:
1936ApJ....84..517H
5. H0: The incredible shrinking constant, 1925-1975 - Virginia
Trimble
Bibcode: 1996PASP..108.1073T
6. Dipole Anisotropy in the COBE Differential Microwave Radiometers
First-Year Sky Maps Kogut. A. at al.
Bibcode: 1993ApJ...419....1K
7. Effects of Red Shifts on the Distribution of Nebulae Hubble Edwin
Bibcode: 1936ApJ....84..517H
8. The 200-inch telescope and some problems it may solve Edwin Hubble
Bibcode: 1947PASP...59..153H
9. The Apparent Anomalous, Weak, Long-Range Acceleration of Pioneer 10
and 11 - Slava G. Turyshev
at al. gr-qc/9903024
10. Indication, from Pioneer 10/11, Galileo, and Ulysses
Data, of an Apparent Anomalous, Weak, Long-Range Acceleration - John D.
Anderson at al. gr-qc/9808081
If there are any comments, please send them to: allen_liou@hotmail.com
